Intermediate Guide
3. Magnitudes
The system that we use for classifying stars in terms of their brightness dates back centuries. The magnitude system was devised around 120 BC by the Greek astronomer Hipparchus who catalogued the stars that he could observe and grouped them by brightness. The brightest stars in the sky would be "of the first magnitude," the next brightest "of the second magnitude," and so on with the dimmest stars he could see labeled as magnitude six. Subsequent inventions showed that there were dimmer objects in the night sky than could be seen with the eye alone, but how to classify them? The magnitude system had to be expanded. It wasn't until the 19th century that technology enabled us to actually measure a star's brightness. Using this innovation, it was determined that a star of the first magnitude was not six times brighter than a star of the sixth, but in fact 100 times brighter. This means that the difference between two adjacent magnitudes is about 2.512 times, and that this is not simply accrued or added up. So how do you calculate how much brighter one star is than another?
More on... Magnitudes
|
Using the example of two stars with magnitudes 1 and 6:
2.5126-1 = 2.5125 = 2.512*2.512*2.512*2.512*2.512 = 100 times
Another example: A magnitude 3 star versus a magnitude 9 star:
2.5129-3 = 2.5126 = 2.512*2.512*2.512*2.512*2.512*2.512 = about 250 times brighter
With more advanced technology also came the ability to measure the magnitude of even brighter objects, such as the star Sirius (magnitude -1.4), the Moon (magnitude -12.6) and the Sun (magnitude -26.7). More recent technology has allowed us to view even fainter deep sky objects (magnitude 28). The Sun is about 7,604,559,076,094,497,624,030 (2.51228-(-26.7) = 2.51254.7 = 7.6x1021) times brighter than these distant objects.
Okay, magnitudes for stars and star-like points such as asteroids are easy enough because they are point sources. Determining the magnitude of other more diffuse objects like nebulae, galaxies, or comets is tougher because their light is spread out in space. Some comets do appear point-like through a telescope because they are either far away enough from Earth so that they just look tiny or far enough from the sun so that we are just seeing the bare nucleus. Then it's easy to compare magnitudes of the comet to nearby stars. But once the comet gets close enough to the sun, then the coma (a huge gas and dust cloud) starts to form and hides the nucleus. But we can still collect magnitudes of the coma, particularly through different filters, to get an idea of how much gas and dust are in the coma.
But what is the magnitude of Tempel 1?
As the comet gets closer to the sun, its coma gets bigger as gas and dust escape the comet and it reflects more light so the comet is brighter. At the same time, the distance between the comet and Earth decreases, so that also makes the comet seem brighter. The comet is closest (~0.71AU or 106 million km)) to Earth when it is at opposition which will be in early May 2005. After that, the Earth will be moving ahead of and away from the comet so that by the July encounter, the comet is about 0.89AUs away. Based on the past behavior of Tempel 1 and taking into account the distances between the comet, sun and earth, a formula can be derived that 'predicts' the brightness.
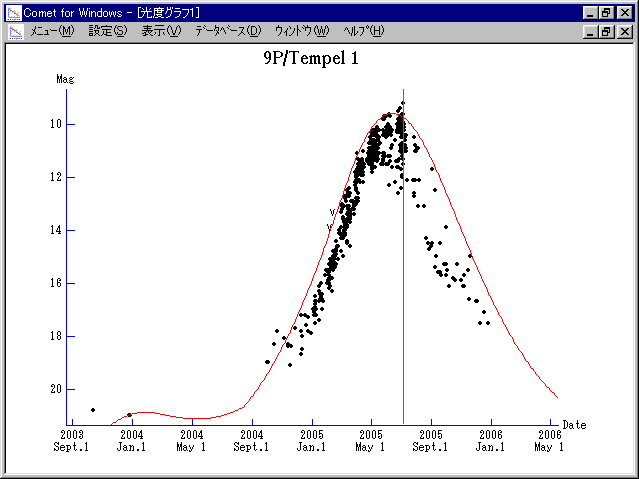
Fig 1: Graph by Seiichi Yoshida
Tempel 1 typically only reaches magnitude 10 or 9 on most favorable apparitions like the one in 2005. The Deep Impact team expects the comet to brighten to 6th or even 5th magnitude when they hit the comet July 4, 2005, one day before its perihelion. The red line is the predicted light curve, the violet vertical line is the comet's perihelion date, and the black dots are observations reported to the MPC.
The above graph is based on the formula 'm1 = 5.5 + 5 log delta + 25 log r' (where m1 = total visual magnitude, delta = earth-comet distance, and r = sun-comet distance) that is used by the Minor Planet Center (MPC) to calculate the brightness of a comet. That formula describes a rapid brightening and fading around the perihelion passage and works best near perihelion. To describe the brightness when Tempel 1 is much further away Mr. Yoshida looked at previous observations and modified the formula to better describe the left portion of the curve. The points along the graph represent some of the observations of Tempel 1 that have been reported to the MPC and were published in an MPEC. Some of the early observations are listed below:
Date Mag. Source Observatory -------------------------------------------------------- 2003 Oct. 12.61361 20.8 MPEC 2004-A17 568 2003 Dec. 25.95785 21 MPEC 2004-A17 461 2003 Dec. 26.91853 21 MPEC 2004-A17 461 2004 Oct. 2.20748 19.0 MPEC 2004-T51 J87 2004 Oct. 4.17592 19.0 MPEC 2004-T51 213
(Please download the MPECs at: cfa-www.harvard.edu/mpec/RecentMPECs.html)
Notice how the dots are very near the line. It will be interesting to watch over the coming months as more data points are added to see how the prediction matches what is observed. What will really be cool is to see the deviation from the prediction after the impactor hits the comet!
Activity
You can make your own graph by
+ generating an ephemeris which includes the magnitude and copying the data into a spreadsheet program (like Excel)
+ or use the formula 'm1 = 5.5 + 5 log delta + 25 log r' to generate the predicted light curve.
Then every couple of days, check out the latest MPECs for reports of observations of Tempel 1 (it will be listed as 9P) and the reported magnitudes and plot them on your graph.
More difficult is to observe Tempel 1 on a regular basis yourself and estimate its magnitude from your observations.
Perihelion occurs on July 5 (the vertical pink line) but Deep Impact hits the comet on July 4.


